Many-Body Simulations and Machine Learning

How can we use complex, dynamical and non-equilibrium many-body simulations for machine learning?
In my PhD project I explore this interdisciplinary research question.

Link Tree

Click here to connect with me and learn more about the Klopotek group. You can contact me directly here.

Videos

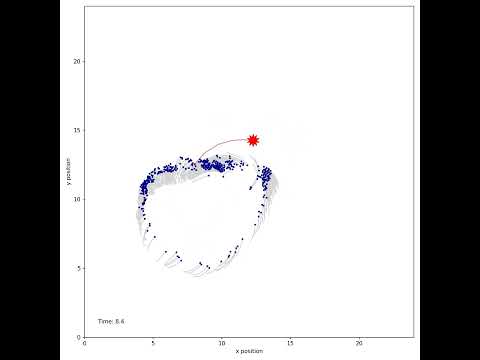
Droplet Phase

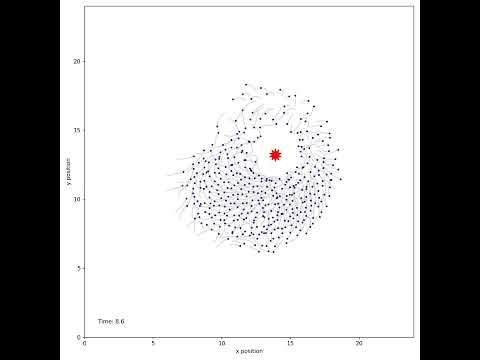
Critical Phase

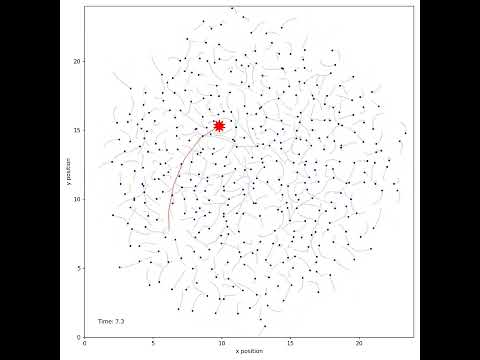
Gas Phase

Poster “The Physics of Reservoir Computing With Swarms”

Presented at:

• SimTech Annual Meeting “StatusSeminar” 2023, Bad Boll, Germany, July 2023
• Workshop “Begegnungszone: Statistical Physics and Machine Learning”, Leipzig, Germany, September 2023

Image Credits and References:

• Lymburn, T., Algar, S. D., Small, M., & Jüngling, T. (2021). Reservoir computing with swarms. Chaos, 31(3), 033121. https://doi.org/10.1063/5.0039745
• Rahman, A., Singwi, K. S., & Sjölander, A. (1962). Theory of Slow Neutron Scattering by Liquids. I. Physical Review, 126(3), 986. https://doi.org/10.1103/PhysRev.126.986
• Kanaya, T., Tsukushi, L., & Kaji, K. (1997). Non-Gaussian Parameter and Heterogeneity of Amorphous Polymers. Progress of Theoretical Physics Supplement No. 126. https://doi.org/10.1143/PTP.126.133/1944200
• Attanasi, A., Cavagna, A., del Castello, L., Giardina, I., Melillo, S., Parisi, L., Pohl, O., Rossaro, B., Shen, E., Silvestri, E., & Viale, M. (2014). Collective Behaviour without Collective Order in Wild Swarms of Midges. PLOS Computational Biology, 10(7), e1003697. https://doi.org/10.1371/JOURNAL.PCBI.1003697
• Gauthier, D. J., Bollt, E., Griffith, A., & Barbosa, W. A. S. (2021). Next generation reservoir computing. Nature Communications, 12(1), 1–8. https://doi.org/10.1038/s41467-021-25801-2
• Soh, H., & Demiris, Y. (2015). Spatio-temporal learning with the online finite and infinite echo-state Gaussian processes. IEEE Transactions on Neural Networks and Learning Systems, 26(3), 522–536. https://doi.org/10.1109/TNNLS.2014.2316291

Poster “Reservoir Computing With Many-Body Simulations”

Presented at:

• SimTech Annual Meeting “StatusSeminar” 2022, Bad Boll, Germany, June 2022
• Bad Honnef DPG Physics School “Deciphering with Chaos: Modeling and understanding complex phenomena with Chaos Theory”, Bad Honnef, Germany, August 2022
• Arnold Sommerfeld School “Physics meets Artificial Intelligence”, Munich, Germany, September 2022
• IMPRS-IS Annual Meeting “Boot Camp” 2022, Blaubeuren, Germany, September 2022
• PLANCKS 2023, Milan, Italy, May 2023

Image Credits and References:

• Gauthier, D. J., Bollt, E., Griffith, A., & Barbosa, W. A. S. (2021). Next generation reservoir computing. Nature Communications, 12(1), 1–8. https://doi.org/10.1038/s41467-021-25801-2
• Lymburn, T., Algar, S. D., Small, M., & Jüngling, T. (2021). Reservoir computing with swarms. Chaos, 31(3), 033121. https://doi.org/10.1063/5.0039745
• Tanaka, G., Yamane, T., Héroux, J. B., Nakane, R., Kanazawa, N., Takeda, S., Numata, H., Nakano, D., & Hirose, A. (2019). Recent advances in physical reservoir computing: A review. In Neural Networks (Vol. 115, pp. 100–123). Pergamon. https://doi.org/10.1016/j.neunet.2019.03.005
• Klopotek, M., Hansen-Goos, H., Dixit, M., Schilling, T., Schreiber, F., & Oettel, M. (2017). Monolayers of hard rods on planar substrates. II. Growth. Journal of Chemical Physics, 146(8), 84903. https://doi.org/10.1063/1.4976308
• Pinna, D., Bourianoff, G., & Everschor-Sitte, K. (2020). Reservoir Computing with Random Skyrmion Textures. Physical Review Applied, 14(5), 054020. https://doi.org/10.1103/PhysRevApplied.14.054020
• Saiki, T. (2017). Switching of localized surface plasmon resonance of gold nanoparticles using phase-change materials and implementation of computing functionality. Applied Physics A: Materials Science and Processing, 123(9). https://doi.org/10.1007/s00339-017-1185-x
• Icons: UXwing.com. https://uxwing.com/license/